本文共 1688 字,大约阅读时间需要 5 分钟。

前言
金三银四,又是一年校招季。
经历过,才深知不易。最近,和作为校招面试官的同事聊了聊,问他们是如何去考察一个学生的,我简单归为以下几点:
- 聪明、反应快,这点自不必说,聪明意味着学习能力、适应力强,能够快速胜任工作。
- 算法不错,代码基本功好,这点其实考察的是算法能力和代码是否写得优雅。
- 基础过硬,技术岗面试最核心的还是考察「技术储备」,包括了语言基本功,操作系统、网络、体系结构、系统设计。
- 语言组织和表达能力,这点很重要,很多同学懂得某个知识点,却很难用简洁准确的语言表述出来。
想必有很多同学在刷题、刷面经,不过我想说“面经虽好,不要贪杯哦~”,面经可以刷,看看面试官都是怎么提问的,但不要寄希望于原题。
因为面试过程中的问题往往是一环扣一环的,这意味着你需要有足够的技术深度,将知识由点连接成面,而不是停留在相互孤立的知识点上。
所以还是建议系统性的看书,如果觉得时间不够,可以关注文章里的重点内容。
接下来是我历时几个月整理出来的Android高级开发面试题以及答案 整理,里面都是一些面试高级工程师必备的知识点,比如:Handler、Activity、Fragment、Service、Android 布局优化、BroadcastReceiver、AsyncTask、Android 事件分发机制、Android View 绘制流程、ANR、Android 内存相关、Android 屏幕适配等等。
接下来我们来看看这些知识点在面试中那些大厂的面试官是怎么问的?自己又能回答多少出来呢?(有些问题不知道答案的这里由于篇幅原因不方便把答案全部放出来,但是答案我都整理成了一份文档文末可以自行领取~)
正文
Android行业主要问题是初级Android太多了,会写xml和Activity的程序员太多了,初中级程序员面临很大的竞争,现状也就偏于高级开发者。越来越多的初中级Android程序员找不到满意的工作,甚至根本找不到工作!所以很多人觉得Android要凉了,甚至不惜转行,从头学其它技术。
现在的Android招聘要求,已经不是多年之前的要求了。
Android 应用开发发展到今天,已经成为一个非常成熟的技术方向,从目前的形势来看,Android开发相当火热,但是高级Android开发人才却比较少,现在移动互联网的开发者也逐步开始关注插件化技术、性能优化、行业新技术,系统架构等进阶技术。
这也同时造成了一种现像,安卓开发人员感受到行业现在已经是冰火两重天的环境了,技术不全面,还停留在中低端技术水平的,辛辛苦苦写代码,为了一年涨2千块钱的工资,疯狂加班,还要被降薪,还要时刻担心被裁员,而中高级开发人员却是企业青睐的对象,工资越来越高,同时也出现了市场岗位情况是中低端开发人员多人竞争一个岗位,而中高级开发人员一人竞争多个岗位的现状。

要如何成为一名Android移动互联网架构师?
针对大家的现状,也为帮助大家能在较短时间内提升自己,今天,就为大家整理分享一份腾讯T3级Android高级技术大纲及课程,文末附有学习资料
Android架构师专题

Android底层开发工程师

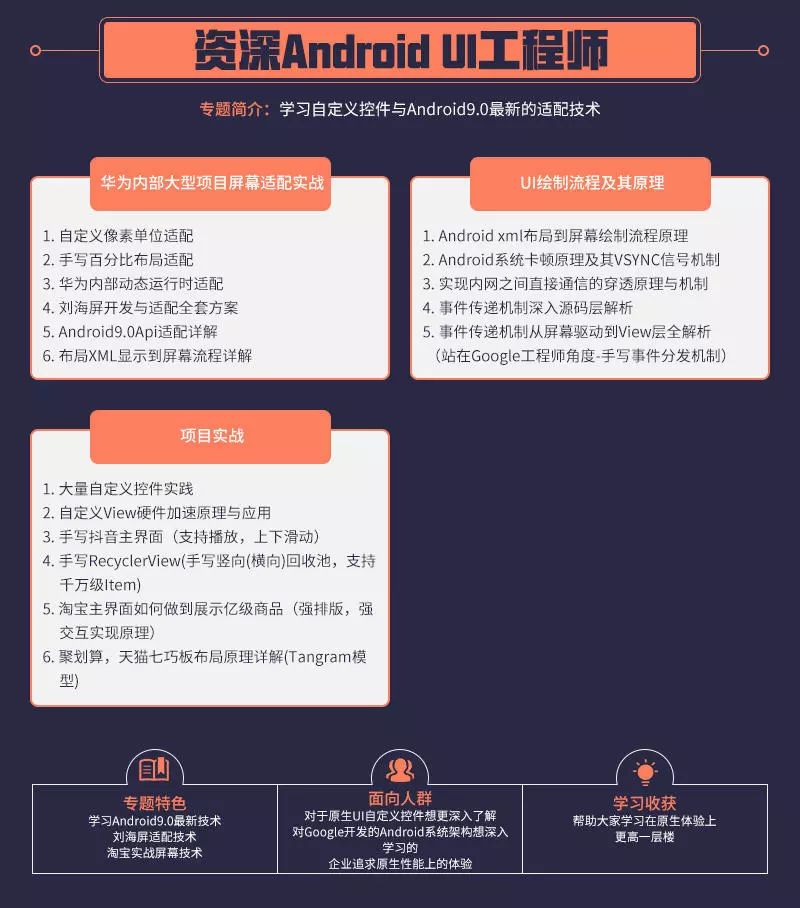
资深Android UI工程师
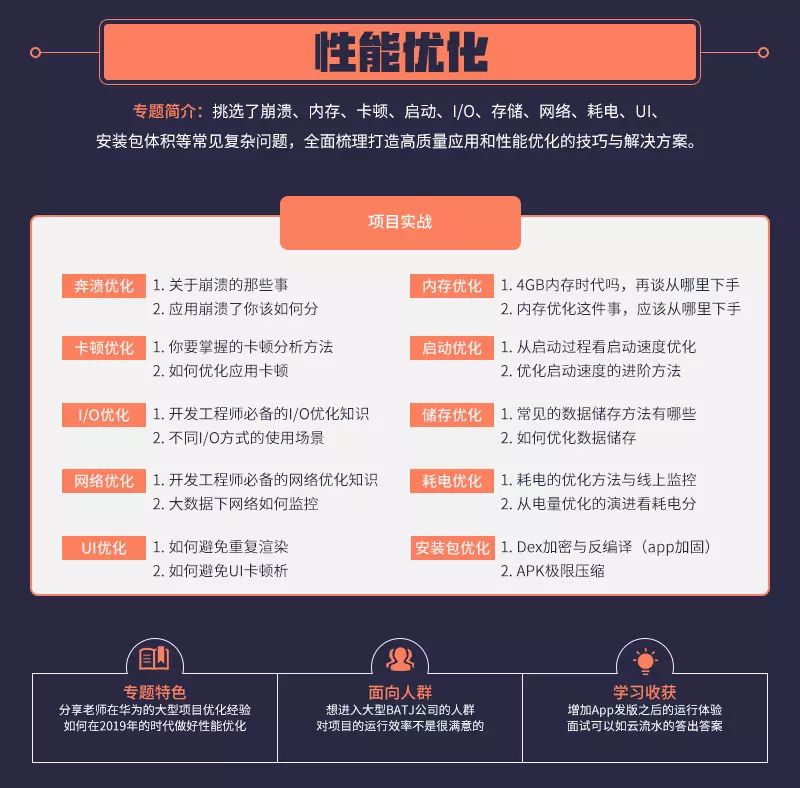
Android必备性能优化
混合式开发工程师

总结
其实要轻松掌握很简单,要点就两个:
- 找到一套好的视频资料,紧跟大牛梳理好的知识框架进行学习。
- 多练。 (视频优势是互动感强,容易集中注意力)
你不需要是天才,也不需要具备强悍的天赋,只要做到这两点,短期内成功的概率是非常高的。
对于很多初中级Android工程师而言,想要提升技能,往往是自己摸索成长,不成体系的学习效果低效漫长且无助。下面资料部分截图是我花费几个月时间整理的,诚意满满:特别适合有3-5年开发经验的Android程序员们学习。
- 自行下载直达领取链接:【】
- 以上进阶BATJ大厂学习资料可以免费分享给大家,需要完整版的朋友,【】。
com/doc/DSkNLaERkbnFoS0ZF)】。**
[外链图片转存中…(img-q3sDxNeB-1618294201931)]
转载地址:http://azbl.baihongyu.com/